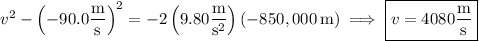Question

A meteorite has a speed of 90.0 m/s when 850 km above the Earth. It is falling vertically (ignore air resistance) and strikes a bed of sand in which it is brought to rest in 3.25 m. (a) What is its speed just before striking the sand?

Answer 1

Before it hits the sand bed, the meteorite is accelerating uniformly with
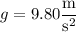`g=9.80(\rm m)/(\mathrm s^2)`, so that its speed
`v` satisfies

`v^2-{v_0}^2=-2g\Delta y`

where
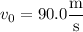`v_0=90.0(\rm m)/(\rm s)` is its initial speed and
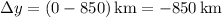`\Delta y=(0-850)\,\mathrm{km}=-850\,\mathrm{km}` is its change in altitude. Notice that we're taking the meteorite's starting position in the atmosphere to be the origin, and the downward direction to be negative. Now,

`v^2-\left(-90.0(\rm m)/(\rm s)\right)^2=-2\left(9.80(\rm m)/(\mathrm s^2)\right)(-850,000\,\mathrm m)\implies\boxed{v=4080(\rm m)/(\rm s)}`