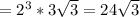Answer:

Explanation:
You need to remember that
![\sqrt[n]{a}](https://img.qammunity.org/2020/formulas/mathematics/middle-school/m5j5nijjmp7ajlqw2bidpiv5jdy1l3j3l3.png) can be written in the following for:
can be written in the following for:
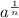
Knowing this and given the expression
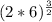 , you need to multiply the numbers inside the parentheses:
, you need to multiply the numbers inside the parentheses:
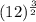
Rewrite it in this form:
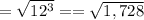
Descompose 1,728 into its prime factors:
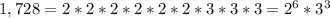
Applying the Product of power property, which states that:
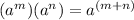
You can say that:
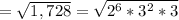
Simplifying, you get: