Question

Express in exponential form.

log(base 2) 16=4

Answer 1

`\log_2 16 = 4 ~~\Longleftrightarrow ~~ 2^4 = 16`

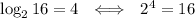
Explanation:

`\log_b x = y ~~\Longleftrightarrow ~~ b^y = x`

`\log_2 16 = 4 ~~\Longleftrightarrow ~~ 2^4 = 16`

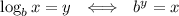
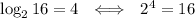
Keep in mind that a log is an exponent.

When you are asked for the log base 2 of 16, you are being asked for the exponent you need to raise 2 to, to get 16. The base in the log is the same base as in the exponential form.

In other words, the question "what is log base 2 of 16?" is the same as the question "what exponent do you raise the base 2 to to get 16?"