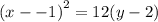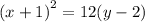Question

If the graph of the following parabola is shifted one unit left and two units up, what is the resulting equation in vertex form? x^2=12

Answer 1

`{(x + 1)}^(2) =12(y - 2)`

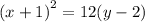
Step-by-step explanation

The original parabola has equation

`{x}^(2) = 12y`

This parabola has its vertex at the origin:

If the parabola is shifted one unit left and two units up, then its new vertex is at (-1,2).

The equation of the new parabola is now of the form:

`{(x - h)}^(2) =1 2(y - k)`

where (h,k) is the vertex.

Substitute the vertex to get:

`{(x - - 1)}^(2) =12(y - 2)`

`{(x + 1)}^(2) =12(y - 2)`