Question

Determine whether the function f(x) = 3(x − 1)4 is even or odd.

Answer 1

The given function is odd

Explanation:

we need to determine the function
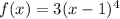`f(x)=3(x-1)^(4)` is odd or even

Since, A function f(x) is said to be even if
`f(-x) = f(x)`

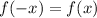
and f(x) is said to be odd if
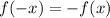`f(-x)= - f(x)` and
`f(-x) \\eq f(x)`

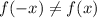
We Replace x with -x in the given function and solve;

`f(x)=3(x-1)^(4)`

`f(-x)=3(-x-1)^(4)`

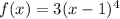
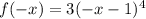
take out the negative common,

`f(-x)=3[-(x+1)]^(4)`

Since
`(-1)^(4)=1`

`f(-x)=3(x+1)^(4)`

`f(-x) \\eq f(x)`

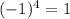
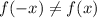
Hence, the given function is odd

Answer 2

the function is odd

Explanation:

A function f(x) is said to be even if f(-x) = f(x)

On the other hand, f(x) is said to be odd if f(-x)≠ f(x).

We plug in -x in place of x in the given function and simplify;

f(-x) = 3(-x-1)^4

f(-x) = 3[-1(x+1)]^4

f(-x) = 3 *(-1)^4 * (x+1)^4

f(-x) = 3(x+1)^4 ≠ f(x)

Therefore, the function given is odd