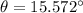Answer:
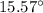
In order to understand better this problem, we can use the figure attached, in which we have a right triangle (with a 90 degree angle), where
 is its hypotenuse,
is its hypotenuse,
 is the adjacent side to the angle of elevation
is the adjacent side to the angle of elevation
 from the boat to the top of the lighthouse and
from the boat to the top of the lighthouse and
 is the opposite side to
is the opposite side to
 .
.
Knowing this, we will use the tangent trigonometric function to find
 :
:
 (1)
(1)
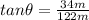 (2)
(2)
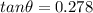 (3)
(3)
 (4)
(4)
Finally: