Answer:
Option C. a = 3.0 cm
Explanation:
We have to find the value of a from the given triangle ABC.
By applying sine rule in ΔABC
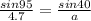
Now we cross multiply in the given equation.
a(sin95°) = 4.7(sin40°)
a(0.9962) = 4.7(0.6428)
a =
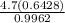
a = 3.03 cm ≈ 3.0 cm
Therefore, a = 3.0cm Option C. will be the answer.