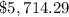Answer:
The minimum amount in sales this month to meet her goal is
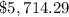
Explanation:
Let
x----> amount in sales this month
we know that
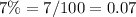
The inequality that represent this situation is equal to
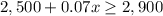
Solve for x
Subtract 2,500 both sides
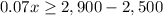
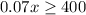
Divide by 0.07 both sides
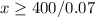

therefore
The minimum amount in sales this month to meet her goal is