Multiplying a vector
 by a constant
by a constant
 gives you a new vector whose components are the same as
gives you a new vector whose components are the same as
 , but scaled by
, but scaled by
 :
:
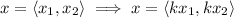
So we have
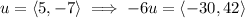
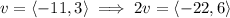
Vector addition is just a matter of adding the corresponding components together:
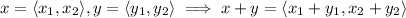
Then
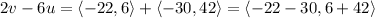
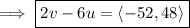
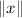 denotes the norm/magnitude of the vector
denotes the norm/magnitude of the vector
 :
:
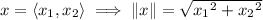
We have

