Question

Suppose that there are two types of tickets to a show: advance and same-day. Advance tickets cost $25 and same-day tickets cost $35. For one performance, there were 45 tickets sold in all, and the total amount paid for them was $1375

. How many tickets of each type were sold?

Answer 1

Answer: 20 advance tickets and 25 same-day tickets.

Explanation:

Set up a system of equations.

Let be "a" the number of advance tickets and "s" the number of same-day tickets.

Then:

`\left \{ {{25a+35s=1375} \atop {a+s=45}} \right.`

You can use the Elimination method. Multiply the second equation by -25, then add both equations and solve for "s":

`\left \{ {{25a+35s=1,375} \atop {-25a-25s=-1,125}} \right.\\.............................\\10s=250\\\\s=(250)/(10)\\\\s=25`

Substitute
`s=25` into an original equation and solve for "a":

`a+(25)=45\\\\a=45-25\\\\a=20`

Answer 2

For this case we propose a system of equations:

x: Variable representing the anticipated tickets

y: Variable representing the same day tickets

So:

`x + y = 45\\25x + 35y = 1375`

We clear x from the first equation:

`x = 45-y`

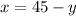
We substitute in the second equation:

`25 (45-y) + 35y = 1375\\1125-25y + 35y = 1375\\10y = 1375-1125\\10y = 250\\y = 25`

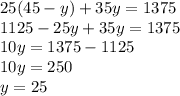
We look for the value of x:

`x = 45-25\\x = 20`

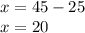
Thus, 20 of anticipated type and 25 of same day type were sold.

Answer:

20 of anticipated type and 25 of same day type were sold.