(a) The pitcher must throw the ball at 27.7 m/s
The momentum of an object is given by:
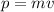
where
m is the mass of the object
v is the object's velocity
Let's calculate the momentum of the bullet, which has a mass of
m = 2.70 g = 0.0027 kg
and a velocity of
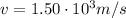
Its momentum is:
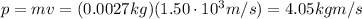
The pitcher must throw the baseball with this same momentum. The mass of the ball is
m = 0.146 kg
So the velocity of the ball must be
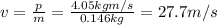
So, the pitcher must throw the ball at 27.7 m/s.
(b) a. The bullet has greater kinetic energy
The kinetic energy of an object is given by

where m is the mass of the object and v is its speed.
For the bullet, we have:
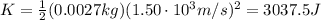
For the ball:
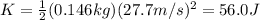
So, the bullet has greater kinetic energy.