I'm guessing the angle is supposed to have measure 60º, not 600º... In this case,
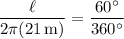
That is, the length of the arc we care about
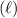 occurs with the circle's circumference in the same ratio as the measure of the central angle it subtends occurs with one complete revolution of the circle. Then with
occurs with the circle's circumference in the same ratio as the measure of the central angle it subtends occurs with one complete revolution of the circle. Then with
 , we have
, we have

so the answer is C.