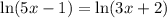
First note that the left side exists only for
 , or
, or
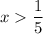 , and the right side exists only for
, and the right side exists only for
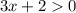 , or
, or
 . Then any solution to this equation is extraneous if we find
. Then any solution to this equation is extraneous if we find
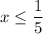 .
.
Write both sides as powers of
 to eliminate the logarithms:
to eliminate the logarithms:
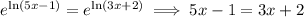
Simplify and solve:
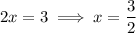
No extraneous solutions here because the one found is larger than 1/5.