Question

The equation for the circle below is x ^2 + y^2 = 100. what is the length of the circles radius?

Answer 1

Answer: 10 units.

Explanation:

The equation of the circle in Center-radius form is:

`(x - h)^2 + (y - k)^2 = r^2`

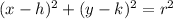
Where the center is (h,k) and "r" is the radius.

The equation of the circle given is:

`x ^2 + y^2 = 100`

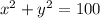
You can observe that is written in Center-radius form.

Then, you can identify that:

`r^2=100`

Knowing this, you need to solve for "r" to find the lenght of the radius.

This is:

`r=√(100)\\\\r=10`

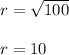
Therefore, the lenght of the radius is 10 units.