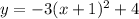Answer:
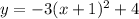
Explanation:
The vertex form of the equation of a parabola is:
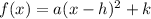
Where (h, k) is the vertex of the parabola.
To write
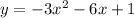 in vertex form, you need to complete the square:
in vertex form, you need to complete the square:
1. Move the 1 to the other side of the equation:
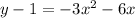
2. Since the leading coefficient must be 1, you need to factor out -3:
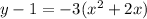
3. Divide the coefficient of the x-term inside the parentheses by 2 and square it:
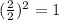
4. Now add 1 inside the parethenses and -3(1) to the other side of the equation (because you factored out -3):
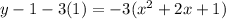

5. Convert the right side of the equation to a squared expression:
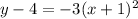
6. And finally, you must solve for "y":