Question

Maths functions
please help!

Answer 1

The equation of f follows the format y=mx+c
You have been given 2 pairs of coordinates that lie on that line
First find the gradient (m) which is equal to change in y / change in x
(4,-1)
(-1,4)
Change in y = -1 - 4 = -5
Change in x = 4 - -1 = 5
Gradient = -5/5 = -1

So now we know y= -x + c
There is only one other unknown so we can sub in a pair of coordinates such as (4,-1)
-1 = -4 + c
Then solve:
Add 4 to both sides
3=c

So the equation of f is y = -x+3
Or f(x)= -x+3

Point a is where this line intersects the x axis, at this point, we know that y=0 which we can sub in
0= -x+3
And solve:
-3 = -x
3=x

So the coordinates of a are (3,0) and because we know that function g is symmetrical in the y axis (due to its form ax^2 + b), the coordinates of c must be (-3,0)

Subbing the coordinates both worked out and given into the function g we get
0 = 9a + b
And
-8 = a + b
Which we can solve simultaneously by subtraction:
0 = 9a + b
-8 = a + b -
——————
8 = 8a

So a = 1
So -8 = 1+b
So -9 = b

So the equation for g is y = x^2 -9

The length of ac is 6 and should be easy to see given the coordinates (3,0) and (-3,0)

The length ob is from the origin (0,0) to the turning point of y = x^2 -9 because the curve is symmetrical and we know it lies on the y axis so x=0 so y = -9

The difference between the coordinates (0,0) and (0,-9) is clearly 9

I hope this helped

Answer 2

`\textsf{1)} \quad f(x)=-x+3`

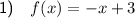
2) A = (3, 0) and C = (-3, 0)

`\textsf{3)} \quad g(x)=x^2-9`

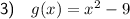
4) AC = 6 units and OB = 9 units

Explanation:

Given functions:

`\begin{cases}f(x)=mx+c\\g(x)=ax^2+b \end{cases}`

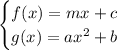
Part (1)

Given points:

• H = (-1, 4)
• T = (4, -1)

As points H and T lie on f(x), substitute the two points into the function to create two equations:

`\textsf{Equation 1}: \quad f(-1)=m(-1)+c=4 \implies -m+c=4`

`\textsf{Equation 2}: \quad f(4)=m(4)+c=-1 \implies 4m+c=-1`

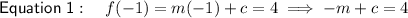
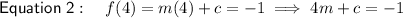
Subtract the first equation from the second to eliminate c:

`\begin{array}{r l} 4m+c & = -1\\- \quad -m+c & = \phantom{))}4\\\cline{1-2}5m \phantom{))))}}& = -5}\end{aligned}`

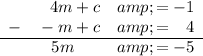
Therefore m = -1.

Substitute the found value of m and one of the points into the function and solve for c:

`\implies f(4)=-1(4)+c=-1`

`\implies c=-1-(-4)=3`

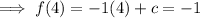
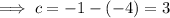
Therefore the equation for function f(x) is:

`f(x)=-x+3`

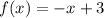
Part (2)

Function f(x) crosses the x-axis at point A. Therefore, f(x) = 0 at point A.

To find the x-value of point A, set f(x) to zero and solve for x:

`\implies f(x)=0`

`\implies -x+3=0`

`\implies x=3`

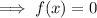
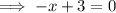
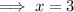
Therefore, A = (3, 0).

As g(x) = ax² + b, its axis of symmetry is x = 0.

A parabola's axis of symmetry is the midpoint of its x-intercepts.

Therefore, if A = (3, 0) then C = (-3, 0).

Part (3)

Points on function g(x):

• A = (3, 0)
• G = (1, -8)

Substitute the points into the given function g(x) to create two equations:

`\textsf{Equation 1}: \quad g(3)=a(3)^2+b=0 \implies 9a+b=0`

`\textsf{Equation 2}: \quad g(1)=a(1)^2+b=-8 \implies a+b=-8`

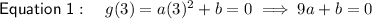
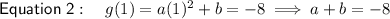
Subtract the second equation from the first to eliminate b:

`\begin{array}{r l} 9a+b & = \phantom{))}0\\- \quad a+b & =-8\\\cline{1-2}8a \phantom{))))}}& = \phantom{))}8}\end{aligned}`

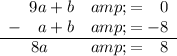
Therefore a = 1.

Substitute the found value of a and one of the points into the function and solve for b:

`\implies g(3)=1(3^2)+b=0`

`\implies 9+b=0\implies b=-9`

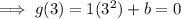
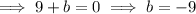
Therefore the equation for function g(x) is:

`g(x)=x^2-9`

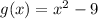
Part 4

The length AC is the difference between the x-values of points A and C.

`\implies x_A-x_C=3-(-3)=6`

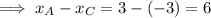
Point B is the y-intercept of g(x), so when x = 0:

`\implies g(0)=(0)^2-9=-9`

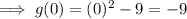
Therefore, B = (0, -9).

The length OB is the difference between the y-values of the origin and point B.

`\implies y_O-y_B=0-(-9)=9`

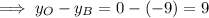
Therefore, AC = 6 units and OB = 9 units