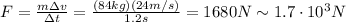Question

William WangHomework #33 Regents Review (3)0989Assignment Mode : Open (Time On Task) 9 of 25 ListenDuring a collision, an 84-kilogram driver of a car moving at 24 meters per second is brought to rest by an inflating air bag in 1.2 seconds. The magnitude of the force exerted on the driver by the air bag is approximately7.0 × 101 N8.2 × 102 N1.7 × 103 N2.0 × 103 NTime on question: 00:02:59

Answer 1

`1.7\cdot 10^3 N`

Step-by-step explanation:

The impulse theorem states that the product between the force and the time interval of the collision is equal to the change in momentum:

`F \Delta t = m \Delta v`

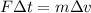
where

F is the force

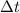`\Delta t` is the time interval

m is the mass

`\Delta v` is the change in velocity

Here we have

m = 84 kg

`\Delta t = 1.2 s`

`\Delta v = 24 m/s`

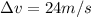
So we can solve the equation to find the force:

`F= (m \Delta v)/(\Delta t )=((84 kg)(24 m/s))/(1.2 s)=1680 N \sim 1.7\cdot 10^3 N`