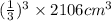ANSWER
The correct answer is C
EXPLANATION
We have that, the smaller cylinder has height 1 cm and the larger cylinder has height 3cm.
The scale factor of similarity is
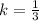
That's if the smaller cylinder is the image.
The volume of the larger cylinder is 2106 cm³.
To find the volume of the smaller cylinder, we multiply the volume of the larger cylinder by k³