Question

A projectile is thrown upward so that its distance above the ground after t seconds is given by the function h(t) = -16t2 + 640t. After how many seconds does the projectile take to reach its maximum height?

Answer 1

20 seconds

Explanation:

We just need the x-coordinate of the vertex...so find -b/(2a) and we are done:

-b/(2a)=-640/(2*-16)=-640/-32=20

20 second

Answer 2

`\boxed{\text{20 s}}`

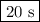
Explanation:

The standard form of a quadratic function is

y = ax² + bx + c

Your function is

h(t) = -16t²+ 640t

a = -16; b = 640; c = 0

a is negative, so you have a downward-opening parabola.

The vertex form of a parabola is

y = a(x - h)² + k

where (h, k) is the vertex of the parabola.

h = -b/(2a) and k = f(h)

Your parabola opens downward, so the vertex is a maximum.

Calculate h

`h = (640)/(-2(-16))} = (640)/(32) = 20\\\text{The projectile reaches maximum height }\boxed{\textbf{20 s}} \text{after it is thrown}\\`

The figure below shows the graph of h(t).