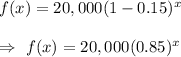Answer:
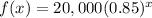
Explanation:
We know that the exponential decay (depreciation) equation with rate of decay r in time period x is given by :-
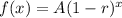 , A is the initial value .
, A is the initial value .
Given: The initial value of truck = $20,000
Rate of depreciation= 15% = 0.15
Now, the function represents the car's value after x years is given by ;-