Question

A flagpole stands in the middle of a flat, level field. 50 feet away from its base, a surveyor measures the angle to the top of the flagpole as 48 degrees. How tall is the flagpole? Round to the nearest hundredth of a foot

Answer 1

55.53 feet.

Explanation:

We have been given that a flagpole stands in the middle of a flat, level field. 50 feet away from its base, a surveyor measures the angle to the top of the flagpole as 48 degrees.

We can see from attached photo that flagpole, the surveyor forms a right triangle with respect to ground.

`\text{tan}=\frac{\text{Opposite}}{\text{Adjacent}}`

`\text{tan}(48^(\circ))=(h)/(50)`

`\text{tan}(48^(\circ))*50=(h)/(50)*50`

`1.110612514829*50=h`

`h=1.110612514829*50`

`h=55.53062574`

`h\approx 55.53`

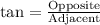
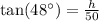
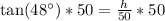
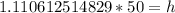
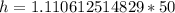
Therefore, the height of the flagpole is approximately 55.53 feet.