Answer:

Explanation:
Given that a cross shaped pattern is made by arranging four identical rectangles around the side of the square . The area of square is 64cm².
So , let's find the side of the square :-
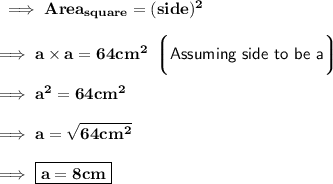
Hence the side of square is 8cm .
Now , breadth of rectangle will be 8cm.
Now its given that the area of rectangle is 1½ times the area of square. So ,

Hence side will be :-

Hence the lenght of rectange is 12cm .
Now , the perimeter of the given figure will be ,

Hence the perimeter of the given cross is 128cm .