Answer:
Explanation:
The goal to solving any equation is to have x = {something}. That means we need to get the x out from underneath that radical. It's a square root, so we can "undo" it by squaring. Square both sides because this is an equation. Squaring both sides gives you

Get everything on one side of the equals sign and set the quadratic equal to 0:
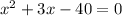
Throw this into the quadratic formula to get that the solutions are x = 5 and -8. We need to see if only one works, both work, or neither work in the original equation.
Does
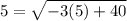 ?
?
 and
and
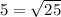
and 5 = 5. So 5 works. Let's try -8 now:
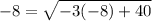 and
and
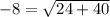 so
so
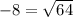
-8 = 8? No it doesn't. So only 5 works. Your choice is the third one down.