Question

JKLM is a rhombus. KM is 20 and JL is 48. Find the perimeter of the

rhombus.

Answer 1

104 units

Explanation:

Given

Shape: Rhombus

`JL = 48`

`KM = 20`

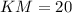
Required

Determine the perimeter

The given parameter are the diagonals of the rhombus.

The perimeter (from diagonals) is calculated as thus:

`P = 2√((JL)^2 + (KM)^2)`

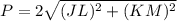
Substitute values for JL and KM

`P = 2√(48^2 + 20^2)`

`P = 2√(2304 +400)`

`P = 2√(2704)`

`P = 2 * 52`

`P = 104`

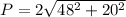
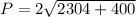
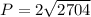
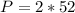
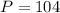
Hence, the perimeter is 104 units