Question

Find the inequality represented by the graph .

Answer 1

The inequality represented by the graph is y ≤
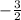`-(3)/(2)` x - 2

Explanation:

The form of the linear equation is y = m x + b, where

• m is the slope of the line

• b is the y-intercept (value y at x = 0)

The rule of the slope is m =
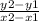`(y2-y1)/(x2-x1)` , where

• (x1, y1) and (x2, y2) are two points on the line

From the given figure

∵ The line passes through points (0, -2) and (2, -5)

∴ x1 = 0 and y1 = -2

∴ x2 = 2 and y2 = -5

→ Substitute them in the rule of the slope above

∵ m =
`(-5--2)/(2-0)` =
`(-5+2)/(2)` =
`(-3)/(2)`

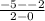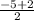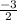
∴ m =
`-(3)/(2)`

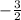
→ b is the value of y at x = 0

∵ At x = 0, y = -2

∴ b = -2

→ Substitute the value of m and b in the form of the equation above

∵ y =
`-(3)/(2)` x + -2

∴ y =
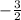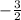`-(3)/(2)` x - 2

∴ The equation of the line is y =
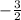`-(3)/(2)` x - 2

∵ The line is solid

∵ The shaded area is under the line

∴ The sign of inequality is ≤

→ Replace = in the equation by ≤

∴ y ≤
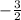`-(3)/(2)` x - 2

∴ The inequality represented by the graph is y ≤
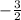`-(3)/(2)` x - 2