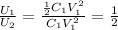Question

Capacitor 2 has half the capacitance and twice the potential difference as capacitor 1.What is the ratio Uc1/Uc2.

Answer 1

1/2

Step-by-step explanation:

The energy stored in a capacitor is given by:

`U=(1)/(2)CV^2`

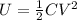
where

C is the capacitance

V is the potential difference

For capacitor 1, we have

`U_1=(1)/(2)C_1V_1^2`

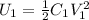
Capacitor 2 has

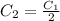`C_2 = (C_1)/(2)` (half the capacitance of capacitor 1)

`V_2 = 2 V_1` (twice the potential difference of capacitor 1)

So the energy of capacitor 2 is

`U_2=(1)/(2)C_2V_2^2=(1)/(2)((C_1)/(2))(2V_1)^2=C_1 V_1^2`

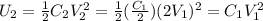
So, the ratio between the two energies is

`(U_1)/(U_2)=((1)/(2)C_1 V_1^2)/(C_1 V_1^2)=(1)/(2)`