Question

Find the area of a regular hexagon inscribed in a circle of radius 6 cm

Answer 1

C

Explanation:

If the hexagon is regular, that means that all of its sides are the same length, all of its interior angles are equal, as are the central angles formed by the triangles within in it. It is one of these triangles that we are concerned about.

If the regular hexagon is inscribed in a circle with a radius of 6, that means that the radius of the hexagon is also 6. The radii of the regular hexagon start at its center and go to each one of the 6 pointy ends (vertices). There are 6 sides so that means that there are 6 radii. That also means that each pair of radii create a triangle. There are 6 triangles inside this hexagon, and all of them are congruent. Because there are 6 central angles and because the degree measure around the outside of a circle is 360 degrees, we can find the vertex angle of each one of these 6 triangles by dividing 360 by 6 to get 60 degrees. The Isosceles Triangle Theorem tells us that if two sides of a triangle are congruent, then the angles opposite those congruent sides are also congruent. So 180 - 60 (the vertex angle) = 120, and 120 divided in half is 60. So this is an equilateral/equiangular triangle. Since all the angles measure 60, that means that all the sides measure the same, as well. So they all measure 6 cm. That gives us that one side of the hexagon measures 6 cm and we will need that for the formula for the area of said hexagon. The altitude of one of those equilateral triangles serves as the apothem that we also need for the area of the hexagon. If we split one of those triangles in half at the altitude, the base will measure 3 and the vertex angle will measure 30 degrees. In the Pythagorean triple for a 30-60-90, the side across from the 30 angle measures x, the side across from the 60 angle measure x times the square root of 3, and the hypotenuse measure 2x. That means that the apothem (which is the altitude of this triangle) is the length across from the 60 angle. So if x measures 3, then the side across from the 60 measures
`3√(3)`

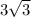
The formula for the area of a regular polygon is

`A=(1)/(2)ap`

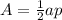
where a is the apothem and p is the perimeter around the hexagon. We found one side to be 6 cm, so 6 times the 6 sides of the hexagon is 36 cm. The apothem is
`3√(3)`

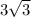
so putting it all together in our formula looks like this:

`A=(1)/(2)(3√(3))(36)`

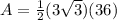
Do the math on that and you will get

`A=54√(3) cm^2`