Question

Several wires of varying thickness are all made of the same material and all have the same length. If the wires are arranged in order of decreasing thickness, what can be said about the ordering of their resistance?

A. The wires will be arranged in order of increasing resistance.
B. The wires will be arranged in order of decreasing resistance.
C. The ordering of the resistances can not be determined because the resistivity is not given.
D. The ordering of the resistances can not be determined because the length is not given.

Answer 1

A. The wires will be arranged in order of increasing resistance.

Step-by-step explanation:

The resistance of a wire is given by

`R=(\rho L)/(A)`

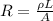
where

`\rho` is the resistivity of the material

L is the length of the wire

A is the cross-sectional area of the wire

In this problem we have several wires made of the same material (so, same
`\rho`) and same length (same L): so, the only quantity that changes is their thickness, so their value of A.

We see from the formula that the resistance R is inversely proportional to the cross-sectional area A: therefore, the smaller the value of A, the larger the value of R. This means that if we arrange the wires in order of decreasing thickness, we are arranging the wires in order of increasing resistance.