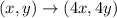Answer: A)
Explanation:
The rule of dilating a shape by scale factor k centered at the origin is given by :-
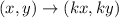 [We multiply k to the coordinates of pre-image ]
[We multiply k to the coordinates of pre-image ]
It means Option C) and D) is incorrect.
From the given graph , it can be observe that quadrilateral R'S'T'U' (image) is larger than the RSTU (pre-image) .
It means the scale factor should be greater than 1. [∵ Scale factor for enlargement is k>1]
Then, Option B is also incorrect.
Hence, the rule yields the dilation of the figure RSTU centered at the origin is given by option A)
i.e.