Suppose θ is an angle in the standard position whose terminal side is in Quadrant IV and . Find the exact values of the five remaining trigonometric functions of θ.

Question

asked Dec 12, 2020 136k views

1 Answer

SubSevn · Answer 1 · 2020-12-18T22:51:04+0000

If
$\theta$ falls in quadrant IV, then we know
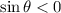$\sin\theta<0$ and
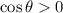$\cos\theta>0$ . By definition of cosecant,

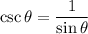
$\csc\theta=\frac1{\sin\theta}$

so we also know that
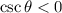$\csc\theta<0$ . Recall that

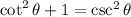
$\cot^2\theta+1=\csc^2\theta$

which means

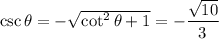
$\csc\theta=-√(\cot^2\theta+1)=-\frac{√(10)}3$

$\implies\sin\theta=-\frac3{√(10)}$

By definition of cotangent,

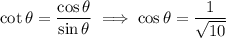
$\cot\theta=(\cos\theta)/(\sin\theta)\implies\cos\theta=\frac1{√(10)}$

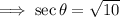
$\implies\sec\theta=√(10)$

We also immediately know that

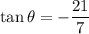
$\tan\theta=-\frac{21}7$

The listed answers are unsimplified relative to the ones we've come up with here, but with some manipulation we find

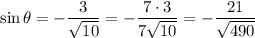
$\sin\theta=-\frac3{√(10)}=-(7\cdot3)/(7√(10))=-(21)/(√(490))$

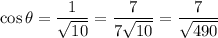
$\cos\theta=\frac1{√(10)}=\frac7{7√(10)}=\frac7{√(490)}$

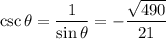
$\csc\theta=\frac1{\sin\theta}=-(√(490))/(21)$

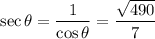
$\sec\theta=\frac1{\cos\theta}=\frac{√(490)}7$

$\tan\theta=\frac1{\cot\theta}=-\frac{21}7$

so that the third option is correct.