Question

Find the area of a triangle with a=20, b=30, and c=40

A.)400.5 units^2
B.)364.0 units^2
C.)290.5 units^2
D.)284.25 units^2

Answer 1

option C 290.5 units^2 is correct.

Explanation:

Answer 2

option C is correct.

Explanation:

The area of triangle with sides a, b and c can be found by using formula

`Area = √(s(s-a)(s-b)(s-c)) \\where \\s= (1)/(2)(a+b+c)`

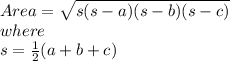
We are given:

a= 20

b=30

c=40

Finding s:

Putting values in the formula and solving:

`s= (1)/(2)(a+b+c)\\s=(1)/(2)(20+30+40)\\s=(90)/(2)\\s= 45`

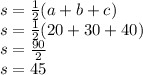
Now, Finding the area:

Putting values in the formula and solving:

`Area = √(s(s-a)(s-b)(s-c))\\Area =√(45(45-20)(45-30)(45-40))\\Area =√(45(25)(15)(5))\\Area = √(84,375)  \\Area = 290.5 units^2`

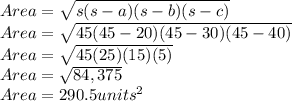
So, option C 290.5 units^2 is correct.