Question 1:
For this case we can raise a rule of three:
63 miles ---------------> 1 hour
189 miles -------------> x
Where "x" represents the number of hours it takes Tanika to travel 189 miles.

So, Tanika takes 3 hours to travel 189 miles
Answer:
Three hours
Question 2:
For this case we must solve the following equations:
A)
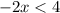
Dividing between -2 on both sides of the inequality:
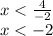
B)
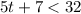
We subtract 7 on both sides of the inequality:
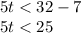
Dividing between 5 on both sides of the inequality:

C)

We subtract 2s on both sides of the inequality:
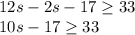
We are 17 on both sides of the inequality:

We divide between 10 on both sides of the inequality:
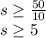
D)
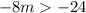
Dividing between -8 on both sides of the inequality:
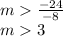
E)
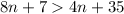
Subtracting 4n on both sides of the inequality:
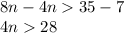
Dividing between 4 on both sides of the inequality:
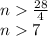
Answer:
