Answer:

Explanation:
Remember:
![(\sqrt[n]{a})^n=a\\\\(a+b)=a^2+2ab+b^2](https://img.qammunity.org/2020/formulas/mathematics/high-school/vtqrju7bwxa5fo101klquo7j084vg0xb6p.png)
Given the equation
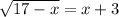 , you need to solve for the variable "x" to find its value.
, you need to solve for the variable "x" to find its value.
You need to square both sides of the equation:
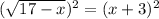
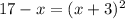
Simplifying, you get:
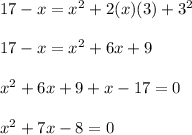
Factor the quadratic equation. Find two numbers whose sum be 7 and whose product be -8. These are: -1 and 8:
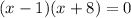
Then:
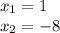
Let's check if the first solution is correct:
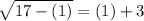
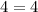 (It checks)
(It checks)
Let's check if the second solution is correct:
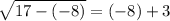
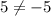 (It does not checks)
(It does not checks)
Therefore, the solution is:
