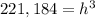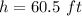Answer:
The height of the water is
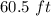
Explanation:
step 1
Find the volume of the tank
The volume of the inverted right circular cone is equal to
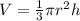
we have
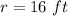
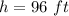
substitute
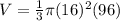
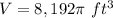
step 2
Find the 25% of the tank’s capacity

step 3
Find the height, of the water in the tank
Let
h ----> the height of the water
we know that
If two figures are similar, then the ratio of its corresponding sides is proportional
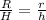
substitute
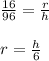
where
r is the radius of the smaller cone of the figure
h is the height of the smaller cone of the figure
R is the radius of the circular base of tank
H is the height of the tank
we have
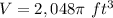 -----> volume of the smaller cone
-----> volume of the smaller cone
substitute

Simplify