Question

12)If p and p' are the length of the perpendiculars drawn from the points (+-root a2-b2,0) to the line x/a cosA + y/b sinA = 1, prove that: p x p' = b2.

Answer 1

Kindly refer to below explanation.

Explanation:

Given equation of line:

`(x)/(a)cos\theta+ (y)/(b)sin\theta =1\\OR\\(x)/(a)cos\theta+ (y)/(b)sin\theta-1=0`

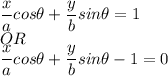
Given points are:

`(√(a^2-b^2),0), (-√(a^2-b^2),0)`

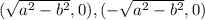
Formula for distance between a point and a line is:

If the point is
`(m, n)` and equation of line is
`Ax+By+C = 0`

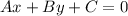
Then, perpendicular distance between line and points is:

`Distance = (|Am+Bn+C|)/(√(A^2+B^2))`

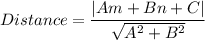
Here,

`A = (x)/(a)cos\theta\\B = (y)/(b)sin\theta\\C = -1`

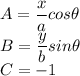
For the first point:

`m = √(a^2-b^2), n = 0`

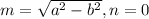
By the above formula:

`p` and
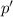`p'` can be calculated as:

`p* p' =\\\Rightarrow \frac{\sqrt{(cos^2\theta)/(a^2)+(sin^2\theta)/(b^2)}}* \frac{\sqrt{(cos^2\theta)/(a^2)+(sin^2\theta)/(b^2)}}\\\Rightarrow (1-(√(a^2-b^2)* (cos\theta)/(a))^2)/((cos^2\theta)/(a^2)+(sin^2\theta)/(b^2))}`

Formula used:

`(x+y)(x-y) = x^2 - y^2`

`\Rightarrow ((a^2-a^2cos^2\theta+b^2cos^2\theta)/(a^2))/((b^2cos^2\theta+a^2sin^2\theta)/(a^2b^2))\\\Rightarrow b^2((a^2sin^2\theta+b^2cos^2\theta)/(a^2sin^2\theta+b^2cos^2\theta))\\\Rightarrow b^2`

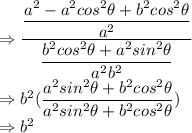
(Using the identity:

`sin^2\theta +cos^2\theta = 1`)

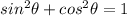(Hence provded)