Because of lack of additional information, let us assume that our horizontal ellipse is centered at the origin. We know that the equation of a horizontal ellipse centered at the the origin is given by:
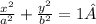
Such that a>b (condition for a horizontal ellipse)
Where a=\frac{1}{2}(major axis)=\frac{1}{2}\times 50=25
Likewise, b=\frac{1}{2}(minor axis)=\frac{1}{2}\times 20=10
Thus, the equation of our horizontal ellipse will be:
\frac{x^2}{25^2} +\frac{y^2}{10^2}=1
Please find the attached file for the graph of our horizontal ellipse.