Question

11. A club has 45 members and its membership committee has 7 members. How many different

membership committees are possible?

Answer 1

45 379 620 ways

Explanation:

45 C 7 = 45! / (7! 38!) = 45379620

Answer 2

Answer:
`\Large\boxed{45379620}`

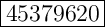
Explanation:

Given information

Total members = 45

Number of membership = 7

Concept

Imagine that the membership is seating where people need to sit:

_____ _____ _____ _____ _____ _____ _____

For every position, there can be only 1 member, and 1 member can only be in one membership position, so there will not be the repetition of members.

Also, since order does not matter in assigning memberships, we use a combination

Given formula

`\dbinom{n}{r}=(n!)/((n-r)!r!)`

`n=size~of~the~set\\r=number~of~selected`

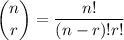
Substitute values into the given formula

`\dbinom{45}{7}=(45!)/((38)!7!)`

Simplify the fraction by canceling out repeating values

`\dbinom{45}{7}=(45*44*43*42*41*40*39*38!)/((38)!7!)`

`\dbinom{45}{7}=\frac{45*44*43*42*41*40*39*\underline{38!}}{(\underline{38)!}7!}`

`\dbinom{45}{7}=(45*44*43*42*41*40*39)/(7!)`

`\Large\boxed{\dbinom{45}{7}=45379620}`

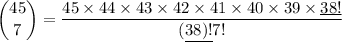
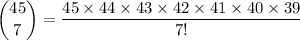
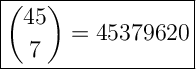
Hope this helps!! :)

Please let me know if you have any questions