Question

Complete the point-slope equation of the line through (1, 3) and (5, 1). Use exact numbers.​

Answer 1

`The \ equation \ in \ point \ and \ slope \ form \ is; \ y - 3 = -(1)/(2) \cdot (x - 1)`

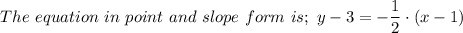
Explanation:

The coordinates of the points through which the line passes are (1, 3) and (5, 1)

The slope, m, of a line given the coordinates of two known points on the line, (x₁, y₁), (x₂, y₂) can be found as follows;

`Slope, \, m =(y_(2)-y_(1))/(x_(2)-x_(1))`

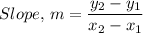
The slope of the given line is therefore
`m =(1-3)/(5-1) = (-2)/(4) = -(1)/(2)`

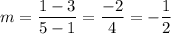
The equation of the line in point and slope form is therefore, y - y₁ = m·(x - x₁) or y - y₂ = m·(x - x₂)

Therefore, by substituting the known values, we have the equation in point and slope form as follows;

`y - 3 = -(1)/(2) \cdot (x - 1)`