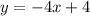Question

find the equation of the line using the slope formula. Write the final equation using the slope-intercept form. the x- intercept is 1, and (x,y) = ( -2, 12) is a point on the line​

Answer 1

`y=- 4x + 4`

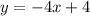
Explanation:

The slope formula for a straight line is:

`y=mx+b`

Where, 'm' is the slope and 'b' is the y-intercept.

To find the x-intercept of a line, we need to equal 'y' to zero, and then solve for 'x'. In this case we know that the x-intercept is 1, so we have the point (x1, y1)=(1,0). We are given a second point which is: (x0, y0)=(-2, 12).

To find the slope, we use the following formula:

`m = (y1-y0)/(x1-x0) = (0-12)/(1-(-2)) = -4`

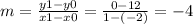
Now, The equation of the line is: y - y0 = m(x-x0). Then, substituting the values of 'm', 'x0' and 'y0' we have that:

`y - 12 = -4(x+2) ⇒ y = -4x-8 + 12 ⇒ y=- 4x + 4`

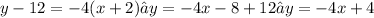
The equation of the line using the slope-intercept form is:

`y=- 4x + 4`