Hello!
The answer is:
The difference between the circle and the square is:
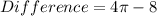
Why?
To solve the problem, we need to find the area of the circle and the area of the square, and then, subtract them.
For the square we have:

We can calculate the diagonal of a square using the following formula:
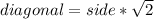
So,
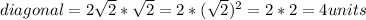
The area will be:
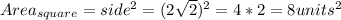
For the circle we have:

The area will be:
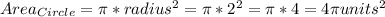
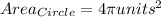
Then, the difference will be:
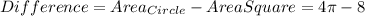
Have a nice day!