Answer:
The trip lasted for a total of 8 hours.
Explanation:
Distance planned = S = 640 miles
Constant speed = V
Thus the time to be taken would be = T = V/S
We have an equation 640 = VT ----- eq (a)
Time For First Quarter = T/4
speed = V
Distance = 640/4 = 160
After first quarter, there is a rest of 1.2 hours and to complete his trip on time, he increased the velocity by 20 mph.
So, the remaining distance = 640 - 160 = 480 miles.
Speed = V + 20 mph
Time remaining = [(T-T/4) - 1.2] = 3T/4 - 1.2 hours
We have an equation for remaining distance s = vt
=> 480 = (V+20)(3T/4 - 1.2) ----- eq (b)
using eq (a), we have V = 640/T. Putting it in eq (b), we have:
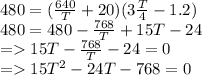
Solving the equation, we get T = 8 or T = -32/5(which is not possible.
So, the right answer is T = 8 hours