Question

If the graph of y= |x| is translated so that the point (1, 1) is moved to (4,1), what is the equation of the new graph?

Answer 1

The new equation is y= (x-3)

Step-by-step explanation: we know that the point (1, 1) is moved to (4,1).

So, the rule of the translation is:
`(x,y) -----> (x+3, y)`

that means the translation is 3 units to the right

Answer 2

`y=|x-3|`

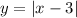
Explanation:

The parent absolute function is

`y=|x|`

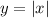
The graph of y= |x| is translated so that the point (1, 1) is moved to (4,1). It means the rule of translation is

`(x,y)\rightarrow (x+3,y)`

it means the graph of y=|x| translated 3 units right. So, the new vertex of the function is (3,0).

The vertex form of an absolute function is

`y=|x-h|+k`

The new vertex of the function is (3,0). Substitute h=3 and k=0 in the above equation.

`y=|x-3|+0`

`y=|x-3|`

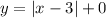
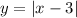
Therefore, the required equation is y=|x-3|.