Question

If a varies directly as b, and a = 28 when b = 7, find b when a = 5.

A 4/5
B 5/4
C 39.2

Answer 1

The correct choice is B.

EXPLANATION

If 'a' varies directly as 'b' then we write the equation of variation,

`a = kb`

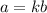
Where k is the constant of variation.

We have that a = 28 when b = 7.

This implies that,

`28= 7k`

`k = 4`

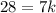
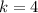
The equation now becomes

`a = 4b`

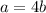
When we a=5, we have

`5 = 4b`

`b = (5)/(4)`

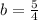
The correct answer is B

Answer 2

Answer: Option B.

`b=(5)/(4)`

Explanation:

If a varies directly with b then when a increases b it also increases by a factor k.

Then we can write that

`a = kb`

We know that when a = 28 then b = 7. So

`28 = k * 7`

`k=(28)/(7)`

`k=4`

Therefore the equation of proportionality is:

`a=4b`

So when
`a=5` then:

`5=4b\\\\b=(5)/(4)`