ANSWER
The correct answer is C
EXPLANATION
The given triangle is a right triangle. Since two angles are equal, it is a right isosceles triangle.
This implies that, x=8 units.
Using Pythagoras Theorem,
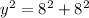
This implies that:
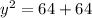
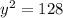
Take positive square root,
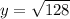

The correct answer is C