Answer:
Option C - Simplify the right side using the "difference of two logs is the log of the quotient" property.
Explanation:
Given : Expression
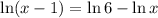
To find : What is the first step in solving the expression ?
Solution :
Expression
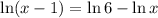
Step 1 - Simplify the right side using the "difference of two logs is the log of the quotient" property.
i.e.

Apply the first step we get,
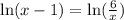
Therefore, Option C is correct.