Question

Use graphs and tables to find the limit and identify any vertical asymptotes of the function.

limit of 1 divided by the quantity x minus 8 as x approaches 8 from the left

Answer 1

it goe towards negative infinity

Explanation:

1/6-8=-1/2

1/7-8=-1

1-7.999-8=-10000

therefore you can assume that it is going to a negative infinity direction

Answer 2

The line x = 8 is our vertical asymptote

The limit of
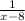`(1)/(x-8)` as x approaches 8 from the left is negative infinity; -∞

Explanation:

We have been given the following function;

`(1)/(x-8)`

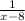
Considering that the function is rational, it will defined everywhere on the real line except where the expression in the denominator will be 0;

That is the function will not be defined where;

x - 8 = 0

solving for x yields;

x = 8

The function will be approaching the vertical line x = 8 asymptotically, meaning that the function will never touch or cross this line. We can therefore say that,

The line x = 8 is our vertical asymptote for the given function

The limit of
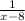`(1)/(x-8)` as x approaches 8 from the left;

`\lim_(x \to8^(-) )(1)/(x-8)`

For x approaching 8 from the left,
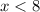`x<8` which implies that
`x-8<0`

The denominator will be a negative quantity approaching 0 from the left, that is -∞.

Thus;

`\lim_(x \to8^(-) )(1)/(x-8)` = -∞

Find the graph attached.