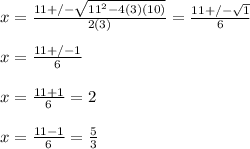Answer:
Part A:
( 1.8333, -0.08333)
Part B:
x = 2 or x = 5/3
Explanation:
The quadratic equation
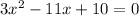 has been given.
has been given.
Part A:
We are required to determine the vertex. The vertex is simply the turning point of the quadratic function. We shall differentiate the given quadratic function and set the result to 0 in order to obtain the co-ordinates of its vertex.
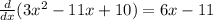
Setting the derivative to 0;
6x - 11 = 0
6x = 11
x = 11/6
The corresponding y value is determined by substituting x = 11/6 into the original equation;
y = 3(11/6)^2 - 11(11/6) + 10
y = -0.08333
The vertex is thus located at the point;
( 1.8333, -0.08333)
Find the attached
Part B:
We can use the quadratic formula to solve for x as follows;
The quadratic formula is given as,
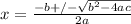
From the quadratic equation given;
a = 3, b = -11, c = 10
We substitute these values into the above formula and simplify to determine the value of x;