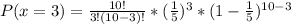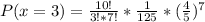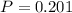Question

In history class, Colin takes a multiple-choice quiz. There are 10 questions. Each question has five possible answers. To the nearest percentage, what is the probability that Colin will get exactly 3 questions correct if he guesses an answer to each question?

Answer 1

Well assuming that the test is a 10 point test. 10 meaning one point each test he would get 30%

Answer 2

`P = 0.201`

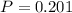
Explanation:

If the discrete random variable X represents the number of correct Colin responses then X can be represented by a binomial distribution with parameters p, n, x.

In this case p represents the probability that colin gets a correct answer, n represents the number of questions.

So the probability that Colin receives x correct questions is:

`P(x) = (n!)/(x!(n-x)!)*p^x*(1-p)^(n-x)`

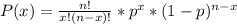
Where:

`p=(1)/(5)`

`n=10`

`x=3`

`P(x=3) = (10!)/(3!(10-3)!)*((1)/(5))^3*(1-(1)/(5))^(10-3)`

`P(x=3) = (10!)/(3!*7!)*(1)/(125)*((4)/(5))^(7)`

`P = 0.201`