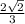Question

24) sin x = 1/3
Find cos x.

Answer 1

`cos(x) =\±2(√(2))/(3)`

Explanation:

We know that
`sen(x) =(1)/(3)`

Remember the following trigonometric identities

`cos ^ 2(x) = 1-sin ^ 2(x)`

Use this identity to find the value of cosx.

If
`sen(x) =(1)/(3)` then:

`cos ^ 2(x) = 1-((1)/(3))^2`

`cos ^ 2(x) =(8)/(9)`

`cos(x) =\±\sqrt{(8)/(9)}`

`cos(x) =\±2(√(2))/(3)`

Answer 2

`(2√(2) )/(3)`

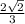
Explanation:

The sine of an angle is defined as the ratio between the opposite side and the hypotenuse of a given right-angled triangle;

sin x = ( opposite / hypotenuse)

The opposite side to the angle x is thus 1 unit while the hypotenuse is 3 units. We need to determine the adjacent side to the angle x. We use the Pythagoras theorem since we are dealing with right-angled triangle;

The adjacent side would be;

`√(9-1)=√(8)=2√(2)`

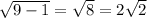
The cosine of an angle is given as;

cos x = (adjacent side / hypotenuse)

Therefore, the cos x would be;

`(2√(2) )/(3)`