For this case we have the following equations:

We must find

By definition of composition of functions we have to:
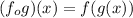
So:
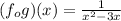
We must find the domain of f (g (x)). The domain will be given by the values for which the function is defined, that is, when the denominator is nonzero.
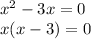
So, the roots are:
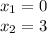
The domain is given by all real numbers except 0 and 3.
Answer:
x other than 0 and 3